Elongated pentagonal gyrobicupola
| Elongated pentagonal gyrobicupola | |
|---|---|
| Type | Johnson J38 - J39 - J40 |
| Faces | 10 triangles 2.10 squares 2 pentagons |
| Edges | 60 |
| Vertices | 30 |
| Vertex configuration | 20(3.43) 10(3.4.5.4) |
| Symmetry group | D5d |
| Dual polyhedron | - |
| Properties | convex |
| Net | |
In geometry, the elongated pentagonal gyrobicupola is one of the Johnson solids (J39). As the name suggests, it can be constructed by elongating a pentagonal gyrobicupola (J31) by inserting a decagonal prism between its congruent halves. Rotating one of the pentagonal cupolae (J5) through 36 degrees before inserting the prism yields an elongated pentagonal orthobicupola (J38).
The 92 Johnson solids were named and described by Norman Johnson in 1966.
Formulae
The following formulae for volume and surface area can be used if all faces are regular, with edge length a:[1]
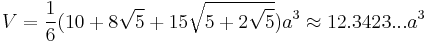
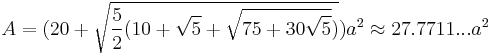
References
- ^ Stephen Wolfram, "Elongated pentagonal gyrobicupola" from Wolfram Alpha. Retrieved July 25, 2010.